Difference between revisions of "Diffraction of Light"
Cmditradmin (talk | contribs) (New page: Return to Basics of Light Menu) |
Cmditradmin (talk | contribs) |
||
| Line 1: | Line 1: | ||
[[Main_Page#Basics of Light|Return to Basics of Light Menu]] | [[Main_Page#Basics of Light|Return to Basics of Light Menu]] | ||
Light can be diffracted | |||
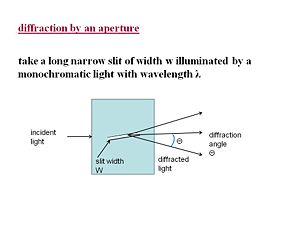
Diffraction effects occur when waves interact with objects having a size similar to the wavelength of radiation. Diffraction is not an important for optical fibers because there must be some specific order in the materials in order to have a diffraction pattern. | |||
When light goes through a slit there are two regimes that have been explored; close to the object as in Fresnel diffraction, and the effects of diffraction far from the object: Fraunhofer diffraction. | |||
The result of diffraction is a set of bright and dark fringes, due to constructive and destructive wave interference, called a diffraction pattern. | |||
[[Image:Diffraction_slit.jpg|thumb|300px|The width of the light and dark lines is depends on the width of the slit and the wavelength of the light.]] | |||
The intensity observed far from the slit is given by: | |||
<math>I = I_0 (\frac {sin\lambda} {\lambda} sinx/x)^2\,\!</math> | |||
where | |||
Where | |||
<math>x = w sin(\frac {\theta}{\lambda})\,\!</math> | |||
minima are found for Θ values such that: | |||
Sin theta min = j (lambda/ w) | |||
with j = ±1, ±2, ±3, … | |||
Therefore: the narrower the slit, the wider the fringe spacing | |||
Revision as of 13:42, 12 May 2009
Return to Basics of Light Menu
Light can be diffracted Diffraction effects occur when waves interact with objects having a size similar to the wavelength of radiation. Diffraction is not an important for optical fibers because there must be some specific order in the materials in order to have a diffraction pattern. When light goes through a slit there are two regimes that have been explored; close to the object as in Fresnel diffraction, and the effects of diffraction far from the object: Fraunhofer diffraction.
The result of diffraction is a set of bright and dark fringes, due to constructive and destructive wave interference, called a diffraction pattern.
The intensity observed far from the slit is given by:
<math>I = I_0 (\frac {sin\lambda} {\lambda} sinx/x)^2\,\!</math>
where Where <math>x = w sin(\frac {\theta}{\lambda})\,\!</math>
minima are found for Θ values such that:
Sin theta min = j (lambda/ w)
with j = ±1, ±2, ±3, … Therefore: the narrower the slit, the wider the fringe spacing