Double Refraction and Birefringence
| Previous Topic | Return to Liquid Crystal Menu | Next Topic |
Wave Surfaces
There are two useful constructs when considering liquid crystals; wave surfaces and index elipsoids.
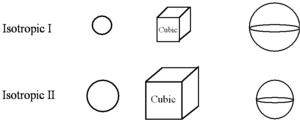
A material that an isotropic refractive index has the same index of refraction from all directions. An example is a crystal with a cubic lattice. The electric field of light is perpendicular to its direction of propagation. A point source emitting light from the center of an isotropic crystal emanates light outward uniformly in all directions. The position of the wave front defines a sphere (wave surface) whose radius is increasing with time
Think about a point source in the center of the material emitting light that forms a wave surface. The wave surface is the tangent to the surface of the wavefront of a wave of light coming from that point. In a isotropic material the wave surface forms a sphere. In a material with a higher index of refraction the wave surface has a smaller radius.
We can also draw another construct with a surface defined by a vector whose magnitude is the refractive index of light travelling in a given direction. In isotropic material this surface would also form a sphere, however since the magnitude of each vector is greater with a higher index of refraction this surface would have a large radius for when there is a higher index of refraction. In the example the first low index material has a larger wave surface than the second material which has a high index.
If we chose another material with a larger susceptibility (more polarizable), its wave surface would expand more slowly because the susceptibility relates to the square of the refractive index. At an arbitrary time (t), the wave surface shows a radius inversely proportional to the index of refraction.
Anisotropic Materials
In an anisotropic material the index of refraction is not the same for each direction. We ask for different polarizations of light what will the wave surface look like?
For non-cubic single crystals, such as a hypothetical crystal of 2,4-hexadiyne, the index of refraction and, hence, the polarizability vary with the direction that the light travels through the crystal.
The hypothetical crystal of 2,4-hexadiyne is uniaxial, where the unique axis is referred to as the optic axis. Light propagates along the optic access and polarization is perpendicular to the direction of propagation. Any direction perpendicular to that axis will have the same index of refraction. If light travels perpendicular to the long axis (eg light is polarized in a different direction) it encounters a lower index of refraction and its wave surface is smaller.
For such a material:
- <math>n_x = n_y ≠n_z\,\!</math>
We can compare two surfaces. The ordinary wave surface (Sord) has a component of polarization that is not along the unique axis (z) of the molecule, that is polarized along Nx or Ny. The extraordinary wave surface (Sext) has a polarization that is oriented along the unique axis (z). This results in a different wave surface depending on the direction. This kind of materials is called birefringent. If you have light shining along the x or y axis travels more slowly resulting in double refraction.
Other non-cubic crystals are characterized by two optical axes (i.e. there are three distinct axes) and are said to be optically biaxial.
| Previous Topic | Return to Liquid Crystal Menu | Next Topic |