Difference between revisions of "Quantum-Mechanical Theory of Molecular Polarizabilities"
Cmditradmin (talk | contribs) m (→Dipole moment) |
Cmditradmin (talk | contribs) m (→Dipole moment) |
||
| Line 9: | Line 9: | ||
=== Dipole moment === | === Dipole moment === | ||
:<math>\ | :<math> \circ \over{A}\,\!</math> | ||
<br clear='all'>[[Image:Dipole_example.png|thumb|300px|]] | <br clear='all'>[[Image:Dipole_example.png|thumb|300px|]] | ||
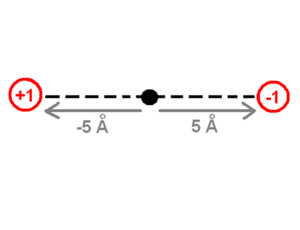
The drawing of the charges shows a point charge of +1 on the left side and a point charge of -1 on the right side. The origin of the system of coordinates is in the middle of these charges. The point charge of +1 is -5 angstrom away from the origin and the point charge of -1 is +5 Angstrom away on the x-axis. The first step to calculate the dipole moment is to multiply the charge +1 by the position -5 Angstrom for the +1 point charge, which will be -5eA (electron times angstrom). Then do the same for the -1 point charge (-1 * 5) which equals -5eA as well. Finally the sum of these values (-5eA + -5eA) -10eA, which corresponds to about -48 debyes, will be the dipole moment. The literature does not use the SI units of the dipole moment because the SI units use coulomb for the charge and meters for the distance which are macroscopic units. Instead, the dipole moment units use electron units and Angstrom (eA), or Debye. | The drawing of the charges shows a point charge of +1 on the left side and a point charge of -1 on the right side. The origin of the system of coordinates is in the middle of these charges. The point charge of +1 is -5 angstrom away from the origin and the point charge of -1 is +5 Angstrom away on the x-axis. The first step to calculate the dipole moment is to multiply the charge +1 by the position -5 Angstrom for the +1 point charge, which will be -5eA (electron times angstrom). Then do the same for the -1 point charge (-1 * 5) which equals -5eA as well. Finally the sum of these values (-5eA + -5eA) -10eA, which corresponds to about -48 debyes, will be the dipole moment. The literature does not use the SI units of the dipole moment because the SI units use coulomb for the charge and meters for the distance which are macroscopic units. Instead, the dipole moment units use electron units and Angstrom (eA), or Debye. | ||
Revision as of 16:11, 18 November 2009
Quantum-Mechanical Theory of Molecular Polarizabilities up to Third Order
The literature refers to first order, second order and third order polarizabilities. You may also see “first order hyperpolarizability “ which is the same thing as second order polarizability. These conventions may be confusing.
Our goal is to be able to relate the chemical structure and the nature of the pi-conjugated backbone, and the nature of donor and acceptors to the to kind of non-linear response that can be measured or calculated.
The expression for dipole moment is simply the sum of all the point charges over all those point charges of the charge itself times the position of that charge.
Dipole moment
- <math> \circ \over{A}\,\!</math>
The drawing of the charges shows a point charge of +1 on the left side and a point charge of -1 on the right side. The origin of the system of coordinates is in the middle of these charges. The point charge of +1 is -5 angstrom away from the origin and the point charge of -1 is +5 Angstrom away on the x-axis. The first step to calculate the dipole moment is to multiply the charge +1 by the position -5 Angstrom for the +1 point charge, which will be -5eA (electron times angstrom). Then do the same for the -1 point charge (-1 * 5) which equals -5eA as well. Finally the sum of these values (-5eA + -5eA) -10eA, which corresponds to about -48 debyes, will be the dipole moment. The literature does not use the SI units of the dipole moment because the SI units use coulomb for the charge and meters for the distance which are macroscopic units. Instead, the dipole moment units use electron units and Angstrom (eA), or Debye.
- <math>Cb \cdot \, m \, or Debye \approx 4.8 q(\vert e \vert) * d( A^\circ )\,\!</math>
If there is a donor acceptor compound, a full charge transfer can occur. The drawing shows the two point charges that are 10 angstrom apart with the length of the conjugated bridge between them. Then with one full electron charge transfer across 10 angstrom, a dipole moment of 50 debyes will be obtained (this is quite large). If the donor and acceptor are about 10 angstrom apart and the dipole moment is about 10 debyes, the system has a charge transfer of about 0.2 electrons from the donor to the acceptor assuming it a simple dipole.
This example is a simplified model where the origin of the system of coordinates was placed midway between the two charges. However, what would happen if the origin of the coordinates is placed on the +1 point charge? What will be the dipole moment? To answer this question, the +1 point charge will be multiplied by a distance of 0 (due to the origin of the coordinates) and that will not contribute anything to the dipole moment. The -1 point charge is now at a distance of 10 angstroms away and is multiplied by a charge of -1 which is -10 eA or about -50 debyes (-48 precisely). Consider the case where the molecule is not neutral but instead a cation is formed with a +2 charge on the left. If the origin of the system of the coordinates is at the +2 point charge, the calculated dipole moment will be -10 eA. But if the origin of system coordinate is placed on the -1 point charge, the dipole moment will be -20 eA. An important lesson out of these examples is that with a charged system, the dipole moment depends the choice of origin of coordinates. The dipole moment is no longer perfectly defined.
In the previous example in which the point origin of coordinates was placed on the +1 point charge, there was a net charge so the monopole is not 0. Therefore, the dipole and all the other poles (quadrapole, octapol, hexapole etc.) depend on the origin of the system of coordinates. However, if the monopole is 0, then the dipole doesn’t depend on the origin of coordinates. But if the dipole is not 0, which means there is a permanent dipole moment in the molecule, then the dipole and the other poles will depend on the origin of coordinates. If the monopole is 0 and the dipole is 0, due to the central symmetric molecule, then the quadrapole will not depend on the choice of the origin of the system of coordinates. It is only when the molecule doesn’t carry a net charge that the dipole is really defined. Otherwise, if the molecule does carry a net charge, the dipole is not defined and it will depend on the origin of the system of coordinates that is chosen. This also creates further complications as it is important to make sure that the point of references is the same for similar systems in order to make comparisons. But for now, when one talks about dipoles, the dipoles will be from neutral molecules so that there will be no complications. In the case of two photon absorption molecules the quadrupole moments can be important. But since these molecules are usually centro symmetric and the dipole is 0, the quadrapole will not depend on the choice of the origin of the system of coordinates.
In the case of point charges where it is easy to describe the degree of dependencies or on the system of coordinates.
- <math>\overrightarrow{\mu} = \sum_i q_i \overrightarrow{r_i}\,\!</math> is the dipole for point charges
But if we have a molecule, the Heisenberg principle does not allow a precise location of the electron. Therefore, wave functions are needed. To get the dipole moment, it is necessary to look at the electronic densities at a given point in space. The expression consists of a wave function at a given point in space r (in other words, wave function at r) and the dipole operator. The dipole operator is the electronic charge of the particles being discussed multiplied by the position of those charges. Since r doesn’t modify Ψ, the Ψ can be arranged so that a psi squared is obtained, which is an electronic density. This makes sense because the wave function squared is an electronic density, and so the expression is equal to an electron density multiplied by a position. Those two expressions are perfectly consistent with one another.
- <math>\overrightarrow{\mu} = \int_{-\infty}^{\infty} \Psi^* e \overrightarrow{r} \Psi d \overrightarrow{r}\,\!</math> is the dipole for a molecule
where
- <math>\Psi^*\,\!</math> is the wavefunction for position r
- <math>e \overrightarrow{r}\,\!</math> is the dipole operator relating the charge and position of charges.
- <math>\langle \Psi \vert \overrightarrow{r} \vert \Psi \rangle\,\!</math> determines the dipole moment in the state described by the wavefunction Ψ
- <math>\overrightarrow{\mu}_g = \langle \Psi_g \vert \overrightarrow{r} \vert \Psi_g \rangle\,\!</math> This is just the Dirac expression for this integral expression.
The dipole moment can be examined at the excited state and this is critical for second order materials. To find the dipole moment in the excited state S1 of organic chromaphore, use the wave function of that particular excited state.