Difference between revisions of "Chromaticity"
Cmditradmin (talk | contribs) |
Cmditradmin (talk | contribs) |
||
| Line 25: | Line 25: | ||
'''z = Z/(X + Y + Z)''' | '''z = Z/(X + Y + Z)''' | ||
Note that x + y + z = 1; thus if two values are known, the third can always be calculated and the z value is usually omitted thus, the x and y values together constitute the chromaticity of a sample light and dark colors that have the same chromaticity (and are therefore plotted at the same point on the two-dimensional chromaticity diagram) can be distinguished in a third dimension (by their luminance or visually perceived brightness). | Note that x + y + z = 1; thus if two values are known, the third can always be calculated and the z value is usually omitted thus, the x and y values together constitute the chromaticity of a sample light and dark colors that have the same chromaticity (and are therefore plotted at the same point on the two-dimensional chromaticity diagram) can be distinguished in a third dimension (by their luminance or visually perceived brightness). | ||
Revision as of 13:25, 4 May 2009
Return to Luminescence Menu | Next Topic
Tristimulus measurement and chromaticity diagrams
The tristimulus color measurement system is based on visually matching a color under standardized conditions against the three primary colors, red, green, and blue; the three results are expressed as X, Y, and Z, respectively, and are called tristimulus values
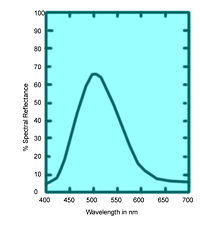
These values specify not only color but also visually perceived reflectance, since they are calculated in such a way that the Y value equals a sample's reflectivity (39.1 percent in this example) when visually compared to a standard white surface by a standard (average) viewer under average daylight.
The tristimulus values of the emerald-green pigment of Figure 6 are X = 22.7, Y = 39.1, and Z = 31.0
The tristimulus values can also be used to determine the visually perceived dominant spectral wavelength (which is related to the hue) of a given sample; the dominant wavelength of the emerald-green pigment is 511.9 nm:
it is based on the values x, y, and z,
Where
x = X/(X + Y + Z)
y = Y/(X + Y + Z)
z = Z/(X + Y + Z)
Note that x + y + z = 1; thus if two values are known, the third can always be calculated and the z value is usually omitted thus, the x and y values together constitute the chromaticity of a sample light and dark colors that have the same chromaticity (and are therefore plotted at the same point on the two-dimensional chromaticity diagram) can be distinguished in a third dimension (by their luminance or visually perceived brightness).
White light is x= 1/3, y = 1/3 and z= 1/3. This is achromatic point. Pure grays and black are the same hue as white light but vary only in the magnitude of their luminance. Occasionally colors will be also be described using luminance as well.
So for the goal of LED makers is to make a white light with x and y values close to 1/3.
Around the horseshoe shaped periphery are the pure saturated colors , beginning with 400nm (violet) and going around to 700 nm (red). Those are the colors of the visible spectrum. The straight line across the bottom are colors that come from the non-spectral mixing of violet and red, they do not correspond to a single wavelength.