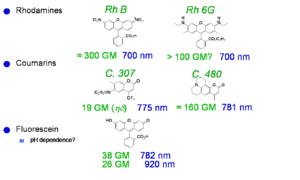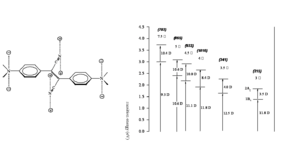Difference between revisions of "Two Photon Absorption"
| Line 47: | Line 47: | ||
<br clear='all'> | <br clear='all'> | ||
=== Two-Photon Processes Provide 3-D Resolution === | === Two-Photon Processes Provide 3-D Resolution === | ||
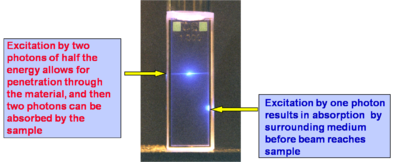
[[Image:Tpa_cuvette_3D.png|thumb|400px|Two laser beams are focused in a sample molecule in solution inside a cuvette; the top | [[Image:Tpa_cuvette_3D.png|thumb|400px|Two laser beams are focused in a sample molecule in solution inside a cuvette; the top beam is tuned at a wavelength at which two-photon absorption can take place, the bottom one at a different wavelength that can excite the molecules by one-photon absorption. The blue light visible in the photograph is the fluorescence emitted by the molecules after excitation.]] | ||
If a light beam is focused into sample at a wavelength suitable for one-photon absorption, molecules are excited throughout the beam path in the sample, as evidenced by the fluorescence emission in the bottom part of the cuvette at right. If the beam is tuned at a wavelength at which the material exhibits two-photon absorption, only the molecules located very close to the focus of the laser beam are excited (top beam in the photograph). This is related to the fact that the excitation rate depends on the square of the light intensity, as discussed above, and that in a focussed beam the light intensity is maximum at the focal plane and decreases approximately with the square of the distance from the focal plane, ''z'', | If a light beam is focused into sample at a wavelength suitable for one-photon absorption, molecules are excited throughout the beam path in the sample, as evidenced by the fluorescence emission in the bottom part of the cuvette at right. If the beam is tuned at a wavelength at which the material exhibits two-photon absorption, only the molecules located very close to the focus of the laser beam are excited (top beam in the photograph). This is related to the fact that the excitation rate depends on the square of the light intensity, as discussed above, and that in a focussed beam the light intensity is maximum at the focal plane and decreases approximately with the square of the distance from the focal plane, ''z'', along the propagation direction (because the area of the beam increases moving away from the focus). Overall, the excitation rate for TPA and the intensity of the two-photon induced fluorescence decrease as the forth power of the distance from the focal plane. Consequently, in the solution in the figure the two-photon induced fluorescence is strongest at the beam focus and its intensity drops off very quickly on either side of the focal plane, resulting in what looks like emission from a "single point" (or small volume) in the solution. At the diffraction limit, the TPA excitation is confined to a volume on the order of the cube of the wavelength of the excitation light. Most of the applications of TPA are based on this ability to confine and control the excitation volume in a material with good resolution in three dimensions, as any excited state process that can take place in the material after two-photon excitation (such as fluorescence emission or energy transfer) will be confined to the same small volume. | ||
=== TPA Processes Provide Improved Penetration of Light Into Absorbing Materials === | === TPA Processes Provide Improved Penetration of Light Into Absorbing Materials === | ||
Revision as of 15:10, 2 June 2010
| Previous Topic | Return to Third-order Processes, Materials & Characterization Menu | Next Topic |
Two-photon absorption (TPA) is a third order nonlinear optical phenomenon in which a molecule absorbs two photons at the same time. The transition energy for this process is equal to the sum of the energies of the two photons absorbed. The probability that a molecules undergoes two-photon absorption depends on the square of the intensity of the incident light, if the two photons are from the same beam (in the case of two incident beams, the transition probability depends on the product of the beams intensities). This intensity dependence is at the origin of the interest in two-photon absorbing materials for use in microscopy and microfabrication applications.
Two-Photon Excited Processes
Two-Photon Absorption
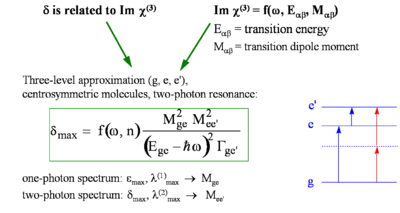
Two-photon absorption in a material can be quantified by the two-photon absorption cross section, a quantity that is proportional to the imaginary component of the χ(3) tensor (or, on a molecular level, to the imaginary component of γ) and depend on the photon energy/energies. In the following we will discuss in detail the case of degenerate (or one-color) two-photon absorption, that is the case where the two photons have the same enrgy. The figure at right schematically illustrates the degenerate two-photon absorption process, in which two photons, each of energy hνA, are simultaneously absorbed and the molecule is excited directly from the ground (S0) state to an excited state (state S2 in the figure) without the formation of an intermediate eigenstate. Neither of the two photons have enough energy to excite the molecule to S2 by itself, but the sum of the energies of the two correspond to the energy of state S2. The dotted line represents a virtual state (as opposed to an actual eigenstate of the system) that can be thought of as created by the absorption of the first photon and having a very short lifetime (on the order of 10-15 sec if the photon energy is not in resonance with any electronic transition). If a second photon is absorbed within this lifetime, the two-photon absorption transition occurs and the molecule is excited to S2.
Once the molecule is in state S2, it quickly relaxes by internal conversion to the lowest excited state (S1 in the figure). From this state, the system can return to the ground state S0 by emission of fluorescence or by nonradiative decay. Alternatively, an additional photon can be absorbed (bringing the molecule to a higher-lying excited state, Sn), or electron or energy transfer to another molecule can take place. Typically, once the molecule is in state S1, the same set of processes will take place whether the molecule was initially excited into S2 by two-photon absorption or directly into S1 by one-photon absorption. For most known materials the two-photon absorption cross section, δ, is small and it is necessary to use intense laser beams in order observe the effects of two-photon absorption.
The rate equation that describes the formation of the excited state of a molecule by one-photon absorption can we written as follows:
- <math>\frac {dN_{OP}} {dt} = \sigma N_{GS} F\,\!</math>
where
- <math>\sigma\,\!</math> is the one-photon absorption cross section (and is related to the transition dipole moment of the molecule between the initial and final states of the transition)
- <math>N_{GS}\,\!</math> is the number of molecules per unit volume in the ground state
- <math>N_{OP}\,\!</math> is the number of molecules per unit volume in the excited state due to one-photon absorption
- <math>F\,\!</math> is the photon flux (number of photons per unit area and time)
- <math>t\,\!</math> is the time
(this is analogous to the equation to describe the rate of a reaction between two reactants, A and B, to give a product C: A + B = C, and that is first-order with respect to each of the reactants).
In a similar manner, the rate equation for two-photon absorption can be written as follows, keeping in mind that two photons are needed to produce one excited molecule (in the reaction analogy, the reaction is now second-order in the photon flux, but still first-order in the concentration of molecules):
- <math>\frac {dN_{TP}} {dt} = \frac {1}{ 2} \delta N_{GS} F^2\,\!</math> is the rate for two photon absorption
where
- <math>\delta\,\!</math> is the two-photon absorption cross section
- <math>N_{TP}\,\!</math> is the number of molecules per unit volume in the excited state due to two-photon absorption
This shows that the probability of two-photon absorption depends on the square of the photon flux (or, equivalently, to the square of the light intensity). The selection rules for the two-photon absorption process are different from those for one-photon absorption (in analogy to the situation for infrared and Raman spectroscopies). In particular, a two-photon absorption transition is allowed only between two states that have the same parity. Thus, in molecules with an inversion center, transitions are two-photon allowed from a gerade (g) state to another gerade state or from an underage (u) to another ungerade state, but not between g and u states. In the case of one-photon absorption, instead, transitions are allowed between an initial state and final state with opposite parity (from g to u, or vice versa). This implies that, in centrosymmetric molecules, if the transition from the ground state to a given excited state is one-photon allowed it is not two-photon allowed and, vice versa, if it is two-photon allowed it is not one-photon allowed. For molecules without inversion center, certain transitions can be both one-photon and two-photon allowed. In the scheme above, the blue arrow represents the excitation of a centrosymmetric molecule to the lowest excited state, S1, by the absorption of one photon (with one-photon absorption cross section σ); this transition is not two-photon allowed. The molecule can be excited by two-photon absorption (red arrows) into a higher-lying state, S2 (with two-photon absorption cross section δ).
Advantages of TPA
Two photon absorption is considered to be a nuisance in the case of all-optical switching using χ(3) materials because it causes attenuation of the light beam and damage to the material can result from severe heating of samples. However the two-photon absorption process can be exploited in another contexts, apart from being of interest in the study of fundamental spectroscopic properties of materials.
Two-Photon Processes Provide 3-D Resolution
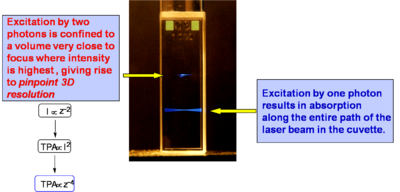
If a light beam is focused into sample at a wavelength suitable for one-photon absorption, molecules are excited throughout the beam path in the sample, as evidenced by the fluorescence emission in the bottom part of the cuvette at right. If the beam is tuned at a wavelength at which the material exhibits two-photon absorption, only the molecules located very close to the focus of the laser beam are excited (top beam in the photograph). This is related to the fact that the excitation rate depends on the square of the light intensity, as discussed above, and that in a focussed beam the light intensity is maximum at the focal plane and decreases approximately with the square of the distance from the focal plane, z, along the propagation direction (because the area of the beam increases moving away from the focus). Overall, the excitation rate for TPA and the intensity of the two-photon induced fluorescence decrease as the forth power of the distance from the focal plane. Consequently, in the solution in the figure the two-photon induced fluorescence is strongest at the beam focus and its intensity drops off very quickly on either side of the focal plane, resulting in what looks like emission from a "single point" (or small volume) in the solution. At the diffraction limit, the TPA excitation is confined to a volume on the order of the cube of the wavelength of the excitation light. Most of the applications of TPA are based on this ability to confine and control the excitation volume in a material with good resolution in three dimensions, as any excited state process that can take place in the material after two-photon excitation (such as fluorescence emission or energy transfer) will be confined to the same small volume.
TPA Processes Provide Improved Penetration of Light Into Absorbing Materials
In a concentrated solution of cumarin one photon absorption (below right) starts immediately at the interface and decays off with the (penetration depth). Because single photons are unable to excite the two photon tuned sample until they are at a critical intensity the two photon laser beam can penetrate farther into a sample. The ability to penetrate a material and to be focused accurately in three dimensions make the TPA ideal for medical applications in which a drug can be activated by TPA at a very precise location.
Calculating and Designing for TPA
Measuring the two photon cross section
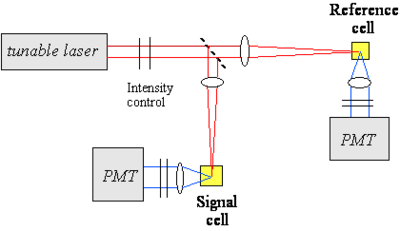
To measure the TPA cross section a tunable laser is focused on a beam splitter which then goes to a reference cell with a photomultiplier tube (PMT) and to a signal cell (with unknown sample) and a PMT. A cross section is created by measuring the fluorescence of the unknown sample across a range of wavelengths produced by a tunable laser. In practice single pulses of intense laser light can vary greatly in intensity, and since TPA is exponentially related to the light intensity these random fluctuations can be exaggerated. By comparing the fluorescence of the sample with each pulse to the reference cell with a known TPA cross section, and then integrating the results you can determine the sample cross section accurately. Otherwise you would have to be able to measure the intensity of each pulse individually which is rather more complicated.
Measurement considerations:
- fs-ns pulse duration; cw
- <math>
n^{(2)}_{ph} = 1/2 \delta(\omega) N F^2(\omega)\,\!</math>
- <math>n_{fl} = \eta n^{(2)}_{ph}\,\!</math>
- only for fluorescent materials
- 90º or backward collection of fluorescence
- <math>signal \propto I^2\,\!</math>
- deviations: linear absorption, stimulated emission, saturation, ...
- importance of spatial and temporal profile
See equipment video on Two-Photon Spectroscopy
Perturbative Expression for γ, as Relevent to Two-Photon Absorption
The perturbative expression for gamma can be applied for two photon absorption with some changes.
- <math>\gamma( -\omega; \omega, -\omega, \omega) \propto \frac{ M^2_{ge} \Delta \mu^2_{ge}} {(E_{ge} - h \omega - i \Gamma_{ge})^2(E_ge-2h\omega -i \Gamma_{ge})} + \frac {M^2_{ge} M^2_{ee^{\prime}}} {(E_{ge} = h\omega- i \Gamma_{ge}) (E_{ge^{\prime}} - 2h\omega - i\Gamma_{ge})}\,\!</math>
The denominator is the difference between the state energy (Ege) and the photon energy (ℏ ω).
There are two components, and the imaginary component accounts for the absorption. The :<math>\Gamma_{ge}\,\!</math> is the damping factor. In order to achieve TPA the sum of the energies of the two photons must equal the energy of the excited state.
For a centrosymmetric molecule :<math>M^2_{ge} \Delta \mu^2_{ge}\,\!</math> (the transition dipole) goes to zero so that entire term goes to zero. For non centrosymmetric molecules both terms are required.
The TPA cross section (δ ) can be related to gamma and several other terms.
- <math>\delta(\omega) = \frac {8\pi^2h\omega^2} {n^2c^2} L^4 Im \gamma( -\omega; \omega, -\omega, \omega)\,\!</math>
Donor-Acceptor TPA Calculations
This is a calculation for a simple donor/acceptor stilbene with an amino group and a formyl group. The delta for the first excited state (S0 to S1) starts out small reaches a peak and then near the cyanine limit it goes down to zero. This is because the delta mu term follows this same pattern.
The change to the second state (S0 to S2) has multiple peaks because the system is two photon resonant and one of the photons is resonant with the S1 state. This is referred to as double resonance and occurs when the transition to S1 is very close to half of the transition to S2. The scale of the cross section for the transition into S1 is on the order 1000 while the transition for S2 is on the order of 10-20000.
See Kogej 1998 [1]
Two-photon absorption example
Here is a centrosymmetric molecule with donor groups on both ends. It has a long conjugation path. There can be excitation into S1, TPA into S2, rapid relaxation by internal conversion back to S1 and then fluorescence coming out of S2. There is no fluorescence from S2 because in most cases the rate of relaxation from S2 to S1 is much faster than the fluorescence lifetime. In fact in centrosymmetric molecules the transition from S2 to S1 is symmetry forbidden for one photon. Therefore the transition dipole moment is close to zero and the coupling between the grounds and the excited state is close to zero resulting in a long radiative lifetime of the excited state. If there is strong transition dipole going up then there will be a short radiative lifetime in the excited state which in turn leads to a high quantum yield for fluorescence. This is because the rate term for this step dominates the other terms in the expression for quantum yield.
However even if the molecule was not centrosymmetric and both one and two photon transitions are possible the internal conversion relaxation is so fast that there still would not be fluorescence from S2. This leads to the rule coined by Michael Kasha that says that no matter what state you excite into fluorescence will only occur from the lowest lying excited state. There are molecules that are exceptions to Kashsa rule such as azuylene but these are very few. The fluorescence from both one and two photon absorption will be from S1.
In the graph note that the energy of the TPA is lower (longer wavelength- red line) than the single photon absorption (blue line) and of the fluorescence (green line). The TPA peak is around 720, dividing this by two the equivalent single photon energy for this would be 360nm. The peak for linear absorption to S1 is 430nm. This shows that each photon in TPA is absorbing to a state that is higher than the S1 state and that there is very little single photon absorption in this zone (the small absorption seen at 360nm is vibrational rather than electronic).
In non linear optics studies it is important to do the measurements at a variety of wavelengths so that you can get a spectrum. You can’t compare molecules by looking at a single wavelength or using the wrong pulse length.
see Rumi 2000 [2]
Laser dyes
Xu and Webb measured the cross section for laser dyes a goupert mayer. One dye had cross section of 300 goupert meyer (two-photon absorptivity, δ, is expressed in Goppert-Mayer units (GM), with 1 GM=1×10-50 cm4 s molecules−1 photon−1.). The process of two photon absorption was predicted by a German physicist Maria Goeppert Mayer in 1929. TPA was not actually observed until the early 60s when lasers were developed that had sufficient intensity to cause it. Most molecules have GM < 1. These dye have very high two photon cross sections..
See Xu and Webb [3] Albota 1998 [4]Fisher 1998 [5]
Vagaries of measurement: The “famous” AF-50
The chart shows various measurements for the molecule AF-50. This is indicative of the problems of measurement in the NLO field. The measurements were made using various techniques and conditions. The experiment with a delta of 11560 was conducted with a timescale of nanoseconds. This is duration is long enough for there to be a convolution of TPA and single photon absorptions that can appear to give a high TPA cross section. This high value could very well be useful for example in making coatings for safety glasses that could exclude high intensity laser light. But it is not useful for the scientific measurement of the molecules.
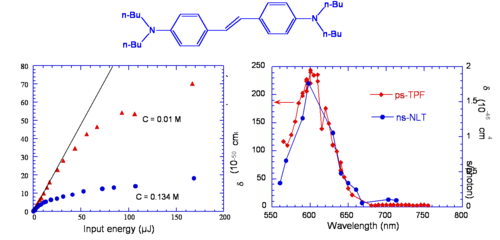
Optical Limiting via Two-Photon Absorption in bis-Donor Stilbene
This molecule behaves as an optical limiter. The curve of two photon fluoresence (TPF shown in red) and non linear transmission (NLT shown in blue) are similar except their scales are different. The NLT scale has units of 10-46 and TPF is on the scale of 200, there is a factor of 100 difference. TPF is measure of the cross section, NLT is an effective cross section that involves two photon absorption followed by excited state absorption.
Initial Observations on Bis-Donor Stilbene
Bis Donor Stilbene (BDAS) is an instructive molecule to study.
Evidence for two-photon absorption
- The molecule is very electron rich
- Strong nonlinear transmission
- Strong blue fluorescence when pumped with orange light
- Fluorescence depends on I2
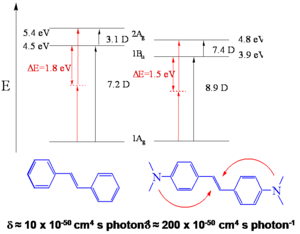
- Two-photon cross section, δ = 210 x 10-50 cm4 s/photon, for stilbene δ = 12 x 10-50 cm4 s/photon
The cross section is about 20 times that for the molecule without the electron donor groups. This is very high value and it is useful to learn why the donors have this effect.
Interesting features for two-photon applications
- High fluorescence quantum yield, φfl ~ 0.9
- High optical transmission
- Low oxidation potential, ED+/D = + 0.035 V vs. Fc/Fc+
It is very easy to oxidize in the ground state and is a powerful reducing agent in the excited state.
Proposed Model to Enhance TPA Cross Sections in Symmetrical Molecules
Theoretical calculations can help to explain this molecule’s properties.
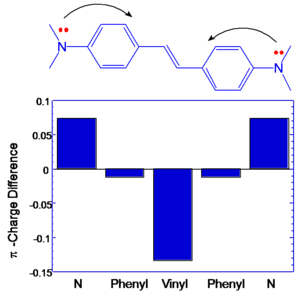
BDAS has large and symmetrical charge transfer from nitrogens (becoming more positive) to central vinyl group in the middle (becoming more negative) that is associated with large transition dipole moment between S1 and S2. (μ ee’2 )
These results suggest that a large change in quadrupole moment between S0 and S1 is leads to enhanced delta. If you want to make dipole (or quadrapole) moment larger you can increase the distance over which charge is separated and you can increase the charge that is separated by that distance, or do both.
Bis Donor Substitution
This suggests certain design strategies for increasing the dipole or quandrupole moment. The transition dipole moment between the ground and first excited state is 7.2 for stilbene and 8.9 for BDAS. Between S1 and S2 the dipole goes from 3.1 D stilbene to 7.4 D for BDAS. This term is squared and this is the reason there such a high TPA cross section for BDAS.
- <math>\delta_{S_0 \rightarrow S_2} \propto \frac {M^2_{ge} M^2_{ee^{\prime}}} {(E_1 - E_0 - \hbar \omega ) \eta} \,\!</math>
Transition Dipole Moments and BOA
If the components that contribute to transition dipole moments are located farther from the middle of the molecule there will be higher transition dipoles. In DBAS large coefficients on nitrogens coupled with extended distance from the molecular origin result in large excited state coupling.
There is an optimal amount of charge transfer that is required to get the largest cross section.
Design of TPA chromophores
To get large quadrupole moment and large quadrupole moment changes you can do the following;
- Increase the distance between the donors so that you increase the distance over which you transfer the charge.
- Increase the strength of the donors so as to increase the amount of charge that is transferred.
- Add acceptors to the middle of molecule so that that section attracts charge.
- Flip the relation with donors in the center and acceptors on the end.
The diagram shows the effect on delta of different donors (shown in blue) and acceptors (shown in red) configurations and longer molecular chain lengths. The combination of these techniques achieves cross-sections in the thousands.
Chain-Length Dependence
The graph shows that as the molecule gets longer the energy of the two photon absorption cross section decreases so the wavelength of the peak increases and the magnitude of the TPA increases due to the increasing transition dipole moments
Transition Dipole Moments
Here is an expression for delta that explores the change in transition dipole moment between the ground state and the excited state and the photon energy. The oscillator strength of the curve is calculated by integrating the absorption spectra plotted in energy and measuring the area under the curve. From this you can extract the transition dipole moment.
- <math>\delta _{max} = f(\omega, n) \frac {M^2_{ge} M^2 _{ee^{\prime}}} {(E_{ge} - \hbar\omega)^2 \Tau_{ge^\prime}}\,\!</math>
where:
- <math>\delta_{max}\,\!</math> is the cross sections that was measured.
- <math>M_{\alpha\beta}\,\!</math> is the transition dipole moment for two levels α,β
- <math>E_{\alpha\beta}\,\!</math> is the energy for absorption to for two levels α,β
- <math>\hbar \omega\,\!</math> is the photon energy that was used to get the TPA.
- <math>\Tau_ge^{\prime}\,\!</math> is the damping term which is estimated
In summary
- <math>\delta_{ge} \propto M^2_{ge} M^2_{ee^\prime} , \Delta E^{-2}\,\!</math>
Effect of D/A Substitution
By making these molecules quadrupolar we increase the transition dipole moment between the first and second excited state (ee and ee’) The data supports this strategy.
Applications for TPA
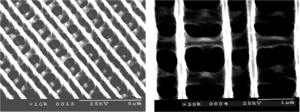
Two-photon initiated polymerization and 3D microfabrication
Two photon absorption can be used to initiate polymerization on a precise microscopic scale. A beam can be focused at 3D coordinates in a polymer causing cross linking. Then non-crosslinked portions are washed away. This can be used for microfabrication. The model of the bull is about 5 microns wide and has some very fine features.
See Wu 1992 [6]
and Maruo 1997 [7]
Two-photon initiators with enhanced sensitivity
Regular photo-initiators are not excellent two photon absorbers. However a femptosecond layer can apply very large power. The goal is strike a power balance between the minimum writing range and the maximum destructive level. For conventional initiators there is only a factor of 2.5 in the power writing range. Newly developed two photon dyes expand this to range to a factor of 50. This lets you write more accurately and faster because the beam does not have remain in the same place as long.
Fluorescent and Refractive Bit Optical Data Storage
This technique can be used to convert non fluorescent polymer into a fluorescent form that has a higher density due to cross linking. If the density goes up and the polarizability stays the same the susceptibility goes up and the refractive index goes up. Peter Renzepus at the UC Urvine is using this method to create 3D optical memory. The ability to write on a 100 different planes increases the amount of information that can be stored (gigabits or terabits of data per cm3)
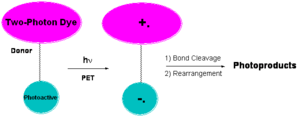
Photochemistry Generated via an Intramolecular Electron Transfer
Another method to initiate this process with electron transfer. A two photon dye connected to a photoactive group will absorb the two photons to cause photoinduced electron transfer (PET) producing a radical anion and radical cation. This group can cleave to give rise to photoproducts. There is a history of doing this kind of chemistry not necessarily with dyes connected to each other and not with two photon absorption.
Why 3D Micro and Nanofabrication
There is a technology pull towards miniaturization of devices and patterned materials.
- Need to free-form fabricate 3 dimensional structures
- Increasing need for ability to pattern a variety of materials
- Need to couple nano-scale object with micro-scale objects
- Areas impacted by 3D micro- and nano-fabrication include MEMs, microfluidics, photonics and tissue engineering. The photo on the left is a chain link fence is twice the thickness of a human hair. In tissue engineering cells grow better on certain topologies (ie scaffolds).
Media: Negative Tone Resist
This is an example of a two photon dye that creates a radical initiator. After absorption the radicals initiate polymerization. The polymer is less soluble and the nonpolymerized material thus this is a known as a negative photo resist.
Sub-diffraction limited resolution
Current lithography techniques are able to make structures at 60 nm. However TPA microfabrication can do this resolution with 3D resolution was well.
Media: Positive Tone Resist
A positive tone resist becomes more soluble in portions that are exposed to light. This allows you to etch away material by exposing it with light.
Tetrahydropyran can be protonated on the oxygen creating a carbo cation thus converting the esther to a carboxylic acid. Esthers are not soluble in basic water but acids are so by dipping the material in an alkaline solution you can dissolve away the exposed material. A proton is needed.
A photo acid generator (PAG) will absorb light and then transfer an electron to the carbon sulfur sigma bond orbital, cause the bond to break homolytically yielding a methyl radical which will attack benzene and create a proton. The proton then starts the esther to acid reaction.
Micro-electromechanical Systems (MEMS) Applications
Micro electro mechanical systems are used for sensors, actuators, micromachines and optical switches. Inkjet heads and disc drive heads are MEMS. These can be fabricated with negative and positive tone materials.
Microscopic Imaging
If you attach a two photon dye to a particular organelle and then scan the cell in 3D with a precise laser beam to build a detailed microscopic 3D model of the structure with submicron resolution. All of this technology begins with the design of molecules that are able absorb light effectively which goes back to third order nonlinear optics, polarizability and hyperpolarizability.
Summary
Perturbation theory predicts which molecules will have large two photon cross sections. Molecules with symmetrical quadrupolar charge transfer lead to large TPA cross sections because they have strong coupling between different excited states. Measurements need to be done very carefully using very short pulses and done over many wavelengths. There are many applications for TPA including microfabrication, optical limiting, and 3D microscopic imaging.
- ↑ T. Kogej et.al. Chem. Phys. Lett. 1998, 298, 1.
- ↑ Rumi et al., JACS 122, 9500, 2000
- ↑ C. Xu, JOSA B, 1996;
- ↑ M. Albota, Appl. Opt., 1998;
- ↑ W. G. Fisher, Appl. Spectr., 1998.
- ↑ E. S. Wu, J. H. Strickler, W. R. Harrell & W. W. Webb, SPIE Proc. 1674, 776 (1992)
- ↑ S. Maruo, O. Nakamura & S. Kawata, Opt. Lett. 22, 132 (1997)